Møbius Donut.

But that’s not all it can do. Microsoft and NASA teamed up to “bring” you, yes you, to Mars.

Follow @the-future-now

But that’s not all it can do. Microsoft and NASA teamed up to “bring” you, yes you, to Mars.

Follow @the-future-now

How does sand from Sahara end up in your windshield ?

TBH cleaning your car is a rather mundane task. But when you fill your head with some interesting physics the task actually gets rather pretty interesting. Here’s some good for thought on such an occasion :

The dust on your windshield might actually be from the Sahara desert

To understand how, lets start with some simple physics.

The stacked ball drop

You basically take couple of balls, align them up and drop them to the ground. The ball at the top reaches the most highest due to the subsequent transfer of energy from the other balls.

                                    Source Video : Physics Girl

Here is an exaggerated but amazing slow motion of the same energy transfer with a water balloon. Notice how the transfer of energy takes place between the water balloon and the tennis ball.

                                     Source Video : Slow Mo Lab

Sandstorms in the desert

Sandstorms/ Dust storms as you might be aware, are pretty common in the desert. . Dust storms arise when a gust front or other strong wind blows loose sand and dirt from a dry surface.

And this can cause something phenomenal to happen:

If the wind speed is sufficient then larger sand particles can propel finer ones high into the atmosphere. ( just like the stacked ball )

Then these fine particles are caught in the global wind pattern and are transported across the globe until they fall down to the earth as rain.

How cool is that ! Have a great day!

* Tracking saharan dust in 3D - NASA video

** All the World’s a Stage … for Dust - NASA article

** Wiki on Saltation

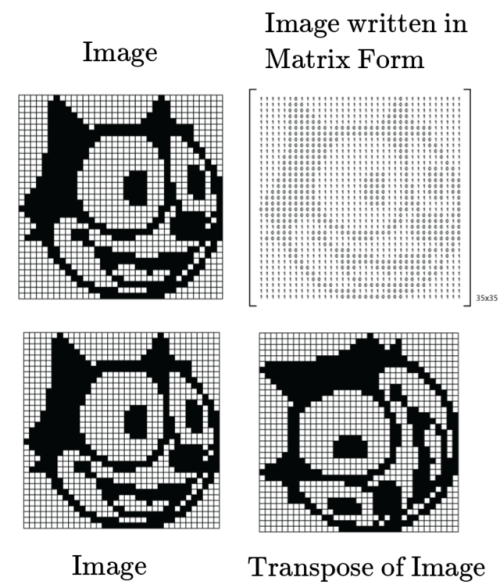
On the transpose of a matrix

In this post, I would just like to highlight the fact an image can be represented in a matrix form and matrix transformations such as transpose, shearing, scaling, etc, from an image processing point of view are purely physical !

Check out this article from the klein project if this post interested you.

Have a great day!

* Interactive Felix the cat and matrix

A sponge can’t soak up mercury. (Video) Facebook | Instagram | Scary Story Website

We started looking at fluctuating loads last time - that is, loads that feature some combination of non-zero mean and alternating stresses - and how to account for them using a Goodman diagram. Let’s re-examine the bracket design problem we did earlier. This time, instead of a fully-reversed load, we’ll assume a fluctuating load with a mean force of 200 lbs, a minimum force of 50 lbs, and a maximum force of 350 lbs. We’ll say the dimensions of the bracket are those we calculated earlier that could handle the fully reversed load. (Problem adapted from Machine Design: An Integrated Approach, 4th Ed., by Robert L. Norton.)

Most of the calculations we did earlier will still hold. We won’t need to recalculate the endurance limit or stress concentration factors. The only new things we need to do are calculate the mean and alternating stresses and the new safety factors.

First step is to calculate the mean and alternating force.

From here, we get the mean and alternating moment.

We’re dealing with a situation of simple bending, so we can calculate mean and alternating stress using the basic bending stress equation.

The geometry of the part hasn’t changed, so we’ll apply the same stress concentration factors that we used before.

Great. We’ve got our new stresses. Now we need to figure out safety factors. As we mentioned earlier, this is now a slightly more complicated proposition. Which safety factor is appropriate will depend on how the alternating and mean stress behave in relation to each other. The possible failure states are shown as points A, B, C, and D on the Goodman diagram for this situation.

We’ll step through all the possible situations one by one using the new stresses we calculated and the endurance limit we got earlier.

Case 1: Constant alternating stress, variable mean stress.

Case 2: Variable alternating stress, constant mean stress.

Case 3: Alternating and mean stress are proportional to each other.

Case 4: Alternating and mean stress vary independently.

We take the worse case, with the failure state F being as close as possible to the current stress situation.

Our design will survive all four cases. Note that Case 4 is always the most conservative case - if you don’t know what your stresses are going to do, this is the one to go with.

Impressive artwork.

Dr. Greg Dunn (artist and neuroscientist) and Dr. Brian Edwards (artist and applied physicist) created Self Reflected to elucidate the nature of human consciousness, bridging the connection between the mysterious three pound macroscopic brain and the microscopic behavior of neurons. Self Reflected offers an unprecedented insight of the brain into itself, revealing through a technique called reflective microetching the enormous scope of beautiful and delicately balanced neural choreographies designed to reflect what is occurring in our own minds as we observe this work of art. Self Reflected was created to remind us that the most marvelous machine in the known universe is at the core of our being and is the root of our shared humanity.

h-t New Scientist: Brain images display the beauty and complexity of consciousness

What is glass?

When most people think of glass, their mind probably jumps straight to windows. And perhaps they’ve heard that old myth - that glass is actually a liquid, not a solid.

So what is glass?

Well, you’ve probably seen something like this before:

The three common phases of matter - gas, liquid, and solid. But you’ll notice that the solid picture is labeled crystalline state. Most people consider glass to be a solid, but it doesn’t quite look like that.

Crystals have a well defined structure, exhibiting long-range order. Glass is what’s called an amorphous material, exhibiting only short-range order. 

Basically, glass is a different kind of solid:

The quartz shown above is an example of a crystalline material. The molecules of glass on the other hand are disordered - yet still solid. 

To create glass, the liquid melt has to be cooled fast enough to prevent the substance from crystallizing. This fast cooling locks the atoms or molecules in the disordered state that looks like the liquid phase. 

Characterizing a substance as a glass also means that this glass transition is reversible. 

While most glass is optically transparent, the properties depend on the composition of the glass. Most of what you see every day is soda-lime-silicate glass, but there are many different kinds of glasses, including sodium borosilicate glass (Pyrex), lead-oxide glass, and aluminosilicate glass.

Sources: x x

PERIODIC SPONGE SURFACES AND UNIFORM SPONGE POLYHEDRA IN NATURE AND IN THE REALM OF THE THEORETICALLY IMAGINABLE

By Michael Burt- Prof emeritus, Technion, I.I.T. Haifa Israel

The diversity of shapes and forms which meets the eye is overwhelming. They shape our environment: physical, mental, intellectual. Theirs is a dynamic milieu; time induced transformation, flowing with the change of light, with the relative movement to the eye, with physical and biological transformation and the evolutionary development of the perceiving mind. “Our study of natural form “the essence of morphology”, is part of that wider science of form which deals with the forms assumed by nature under all aspects and conditions, and in a still wider sense, with forms which are theoretically imaginable…..(On Growth and Form – D'Arcy Thompson), “Theoretically” to imply that we are dealing with causal- rational forms. “It is the business of logic to invent purely artificial structures of elements and relations. Sometimes one of these structures is close enough to a real situation to be allowed to represent it. And then, because the logic is so tightly drawn, we gain insight into the reality which was previously withheld from us” (C. Alexander). A particular interest should be focused on those structures which are shaped like solids or containers, with continuous two-manifold enveloping surfaces, enclosing a volume of space and thus subdividing the entire space into two complementary sub-spaces, sometimes referred to as interior and exterior, although telling which is which, is a relativistic notion. On each of these envelopes, topologically speaking, an infinite number of different maps composed of polygonal regions (faces), which are bounded by sets of edge segments and vertices, could be drawn, to represent what we call polyhedra, or polyhedral envelopes. We come to know them by various names and notations, evolving through many historical cultures up to our present times; each representing an individual figure-polyhedron, or a family, a group, a class or a domain; convex-finite, Platonic and Archimedean polyhedra; pyramids, prisms; anti-prisms; star polyhedra; deltahedra; zonohedra; saddle polyhedra, dihedral, polydigonal, toroidal, sponge like, finite and infinite polyhedra; regular, uniform, quasi-regular, and so forth; all inscribable in our 3-dimensional space. It is these structures and their extended derivatives which shape our physical-natural or artificial man-conceived environment and provide for our mental pictures of its architecture. The number of forms which had acquired a name or a specific notation through the ages is amounting to infinity, although the number of those which comprise our day to day formal vocabulary and design imagery is extremely (and regretfully) limited by comparison, even amongst designers and architects, whose profession, by definition, compels them to manipulate and articulate forms and space. Here it is right to observe that name-giving is part of the creative and generative process. The number of polyhedral forms which did not receive, as yet a proper name or a notation is also infinite. Infinite is also the number of potentially existing and possible imaginary periodic forms, not envisaged yet. Conspicuous are those relating to sponge-like labyrinthian, polyhedral, space dividing surfaces, which until quite recently were not even considered as a research topic. The interest in these forms has been prompted by our growing awareness of their abundance in nature and their importance, not only in describing micro and macro-physical and biological phenomena, but also in coping with morphological complexity and nature of our built environment and its emerging new architecture and the order and formal character of our living spaces, on either the building or the urban scale. Nature is saturated with sponge structures on every possible scale of physical-biological reality. The term was first adopted in biology: “Sponge: any member of the phylum Porifera, sessile aquatic animals, with single cavity in the body, with numerous pores. The fibrous skeleton of such an animal, remarkable for its power of sucking up water”. (Wordsworth dictionary). the entire study here

© Michael Burt- Prof emeritus, Technion, I.I.T. Haifa Israel

Why most metals are silver (but copper and gold aren’t)

If we want to understand what gives a metal its color we first need to understand a little bit about the definition of a metal. Metals are materials that experience metallic bonding - wherein the atoms are so close that there is a veritable “sea of electrons” in the substance. (This is also what makes metals conductors, but that’s another story). Basically each atom donates an electron or two that is free to flow throughout the material, unattached to any particular nucleus. 

This proximity leads to an overlap in the allowed energy levels of electrons (shown in the lower left hand image above); basically the higher empty electronic levels are so close to the uppermost filled levels (also called the Fermi level) that they form an essentially continuous band of allowed energies. 

Now, backtracking a little bit, color in a substance is caused when a material doesn’t absorb a particular wavelength of light. Because of the empty energy levels mentioned above, metals generally can absorb all wavelengths of light in the visible spectrum. This implies that a metal should look black, except that the excited electron can immediately fall back to the state that it came from, emitting exactly the same energy, causing a flat piece of metal to appear reflective. Thus, the reason why most metals are silver. (Also, the flatter a metal, the more reflective, thanks to diffuse vs. specular reflection).

For a few select metals, like copper and gold, the absorption and emission of photons are noticeably dependent on wavelength across the visible part of the spectrum. The graph in the lower right image above shows the reflectance of aluminum, silver, and gold, including wavelengths in the infrared and ultraviolet. Aluminum is pretty reflective overall, and silver is highly reflective in the visible region (about 400 to 700 nm), but gold clearly absorbs wavelengths about 500 nm or below. Thus, it most strongly reflects yellow, giving it its characteristic appearance. 

Sources: (first image), 2 (second image), 3 (third image), 4