Cassini Spacecraft: Top Discoveries
Cassini Spacecraft: Top Discoveries
Our Cassini spacecraft has been exploring Saturn, its stunning rings and its strange and beautiful moons for more than a decade.

Having expended almost every bit of the rocket propellant it carried to Saturn, operators are deliberately plunging Cassini into the planet to ensure Saturn’s moons will remain pristine for future exploration – in particular, the ice-covered, ocean-bearing moon Enceladus, but also Titan, with its intriguing pre-biotic chemistry.
Let’s take a look back at some of Cassini’s top discoveries:
Titan

Under its shroud of haze, Saturn’s planet-sized moon Titan hides dunes, mountains of water ice and rivers and seas of liquid methane. Of the hundreds of moons in our solar system, Titan is the only one with a dense atmosphere and large liquid reservoirs on its surface, making it in some ways more like a terrestrial planet.

Both Earth and Titan have nitrogen-dominated atmospheres – over 95% nitrogen in Titan’s case. However, unlike Earth, Titan has very little oxygen; the rest of the atmosphere is mostly methane and traced amounts of other gases, including ethane.

There are three large seas, all located close to the moon’s north pole, surrounded by numerous smaller lakes in the northern hemisphere. Just one large lake has been found in the southern hemisphere.
Enceladus

The moon Enceladus conceals a global ocean of salty liquid water beneath its icy surface. Some of that water even shoots out into space, creating an immense plume!

For decades, scientists didn’t know why Enceladus was the brightest world in the solar system, or how it related to Saturn’s E ring. Cassini found that both the fresh coating on its surface, and icy material in the E ring originate from vents connected to a global subsurface saltwater ocean that might host hydrothermal vents.

With its global ocean, unique chemistry and internal heat, Enceladus has become a promising lead in our search for worlds where life could exist.
Iapetus

Saturn’s two-toned moon Iapetus gets its odd coloring from reddish dust in its orbital path that is swept up and lands on the leading face of the moon.

The most unique, and perhaps most remarkable feature discovered on Iapetus in Cassini images is a topographic ridge that coincides almost exactly with the geographic equator. The physical origin of the ridge has yet to be explained…

It is not yet year whether the ridge is a mountain belt that has folded upward, or an extensional crack in the surface through which material from inside Iapetus erupted onto the surface and accumulated locally.
Saturn’s Rings

Saturn’s rings are made of countless particles of ice and dust, which Saturn’s moons push and tug, creating gaps and waves.

Scientists have never before studied the size, temperature, composition and distribution of Saturn’s rings from Saturn obit. Cassini has captured extraordinary ring-moon interactions, observed the lowest ring-temperature ever recorded at Saturn, discovered that the moon Enceladus is the source for Saturn’s E ring, and viewed the rings at equinox when sunlight strikes the rings edge-on, revealing never-before-seen ring features and details.
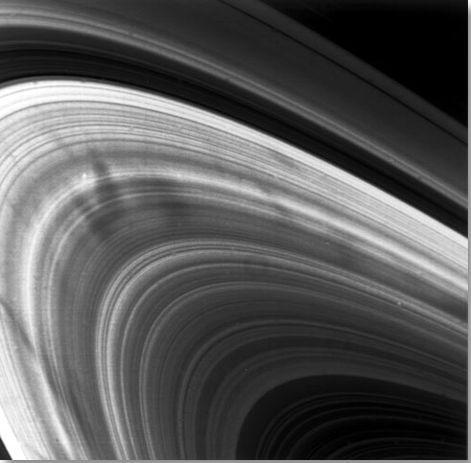
Cassini also studied features in Saturn’s rings called “spokes,” which can be longer than the diameter of Earth. Scientists think they’re made of thin icy particles that are lifted by an electrostatic charge and only last a few hours.
Auroras

The powerful magnetic field that permeates Saturn is strange because it lines up with the planet’s poles. But just like Earth’s field, it all creates shimmering auroras.

Auroras on Saturn occur in a process similar to Earth’s northern and southern lights. Particles from the solar wind are channeled by Saturn’s magnetic field toward the planet’s poles, where they interact with electrically charged gas (plasma) in the upper atmosphere and emit light.
Turbulent Atmosphere

Saturn’s turbulent atmosphere churns with immense storms and a striking, six-sided jet stream near its north pole.

Saturn’s north and south poles are also each beautifully (and violently) decorated by a colossal swirling storm. Cassini got an up-close look at the north polar storm and scientists found that the storm’s eye was about 50 times wider than an Earth hurricane’s eye.

Unlike the Earth hurricanes that are driven by warm ocean waters, Saturn’s polar vortexes aren’t actually hurricanes. They’re hurricane-like though, and even contain lightning. Cassini’s instruments have ‘heard’ lightning ever since entering Saturn orbit in 2004, in the form of radio waves. But it wasn’t until 2009 that Cassini’s cameras captured images of Saturnian lighting for the first time.

Cassini scientists assembled a short video of it, the first video of lightning discharging on a planet other than Earth.

Cassini’s adventure will end soon because it’s almost out of fuel. So to avoid possibly ever contaminating moons like Enceladus or Titan, on Sept. 15 it will intentionally dive into Saturn’s atmosphere.

The spacecraft is expected to lose radio contact with Earth within about one to two minutes after beginning its decent into Saturn’s upper atmosphere. But on the way down, before contact is lost, eight of Cassini’s 12 science instruments will be operating! More details on the spacecraft’s final decent can be found HERE.
Make sure to follow us on Tumblr for your regular dose of space: http://nasa.tumblr.com
More Posts from Jupyterjones and Others
When 25,000 little dice are agitated in a cylinder, they form into neat concentric circles

Behold the magic of compaction dynamics. Scientists from Mexico and Spain dumped 25,000 tiny dice (0.2 inches) into a large clear plastic cylinder and rotated the cylinder back and forth once a second. The dice arranged themselves into rows of concentric circles. See the paper and the videos here.
https://boingboing.net/2017/12/05/when-25000-little-dice-are-ag.html
There are 27 straight lines on a smooth cubic surface (always; for real!)
This talk was given by Theodosios Douvropoulos at our junior colloquium.
I always enjoy myself at Theo’s talks, but he has picked up Vic’s annoying habit of giving talks that are nearly impossible to take good notes on. This talk was at least somewhat elementary, which means that I could at least follow it while being completely unsure of what to write down ;)
——
A cubic surface is a two-dimensional surface in three dimensions which is defined by a cubic polynomial. This statement has to be qualified somewhat if you want to do work with these objects, but for the purpose of listening to a talk, this is all you really need.
The amazing theorem about smooth cubic surfaces was proven by Arthur Cayley in 1849, which is that they contain 27 lines. To be clear, “line” in this context means an actual honest-to-god straight line, and by “contain” we mean that the entire line sits inside the surface, like yes all of it, infinitely far in both directions, without distorting it at all.

(source)
[ Okay, fine, you have to make some concession here: the field has to be algebraically closed and the line is supposed to be a line over that field. And $\Bbb R$ is not algebraically closed, so a ‘line’ really means a complex line, but that’s not any less amazing because it’s still an honest, straight, line. ]
This theorem is completely unreasonable for three reasons. First of all, the fact that any cubic surface contains any (entire) lines at all is kind of stunning. Second, the fact that the number of lines that it contains is finite is it’s own kind of cray. And finally, every single cubic surface has the SAME NUMBER of lines?? Yes! always; for real!
All of these miracles have justifications, and most of them are kind of technical. Theo spent a considerable amount of time talking about the second one, but after scribbling on my notes for the better part of an hour, I can’t make heads or tails of them. So instead I’m going to talk about blowups.
I mentioned blowups in the fifth post of the sequence on Schubert varieties, and I dealt with it fairly informally there, but Theo suggested a more formal but still fairly intuitive way of understanding blowups at a point. The idea is that we are trying to replace the point with a collection of points, one for each unit tangent vector at the original point. In particular, a point on any smooth surface has a blowup that looks like a line, and hence the blowup in a neighborhood of the point looks like this:

(source)
Here is another amazing fact about cubic surfaces: all of them can be realized as a plane— just an ordinary, flat (complex) 2D plane— which has been blown up at exactly six points. These points have to be “sufficiently generic”; much like in the crescent configuration situation, you need that no two points lie on the same line, and the six points do not all lie on a conic curve (a polynomial of degree 2).
In fact, it’s possible, using this description to very easily recover 21 of the 27 lines. Six of the lines come from the blowups themselves, since points blow up into lines. Another fifteen of them come from the lines between any two locations of blowup. This requires a little bit of work: you can see in the picture that the “horizontal directions” of the blowup are locally honest lines. Although most of these will become distorted near the other blowups, precisely one will not: the height corresponding to the tangent vector pointing directly at the other blowup point.
The remaining six points are can also be understood from this picture: they come from the image of the conic passing through five of the blowup points. I have not seen a convincing elementary reason why this should be true; the standard proof is via a Chow ring computation. If you know anything about Chow rings, you know that I am not about to repeat that computation right here.
This description is nice because it not only tells us how many lines there are, but also it roughly tells us how the lines intersect each other. I say “roughly” because you do have to know a little more about what’s going on with those conics a little more precisely. In particular, it is possible for three lines on a cubic surface to intersect at a single point, but this does not always happen.
I’ll conclude in the same way that Theo did, with a rushed comment about the fact that “27 lines on a cubic” is one part of a collection of relations and conjectured relations that Arnold called the trinities. Some of these trinities are more… shall we say… substantiated than others… but in any case, the whole mess is Laglandsian in scope and unlikely even to be stated rigorously, much less settled, in our lifetimes. But it makes for interesting reading and good fodder for idle speculation :)

That’s the secret of programming.






The 21 Card Trick created in Python.
See how it works and read a little more about it here: [x]
Feel free to ask any questions you may have. :)
Regarding Fractals and Non-Integral Dimensionality
Alright, I know it’s past midnight (at least it is where I am), but let’s talk about fractal geometry.
Fractals
If you don’t know what fractals are, they’re essentially just any shape that gets rougher (or has more detail) as you zoom in, rather than getting smoother. Non-fractals include easy geometric shapes like squares, circles, and triangles, while fractals include more complex or natural shapes like the coast of Great Britain, Sierpinski’s Triangle, or a Koch Snowflake.

Fractals, in turn, can be broken down further. Some fractals are the product of an iterative process and repeat smaller versions of themselves throughout them. Others are more natural and just happen to be more jagged.

Fractals and Non-Integral Dimensionality
Now that we’ve gotten the actual explanation of what fractals are out of the way, let’s talk about their most interesting property: non-integral dimensionality. The idea that fractals do not actually have an integral dimension was originally thought up by this guy, Benoit Mandelbrot.

He studied fractals a lot, even finding one of his own: the Mandelbrot Set. The important thing about this guy is that he realized that fractals are interesting when it comes to defining their dimension. Most regular shapes can have their dimension found easily: lines with their finite length but no width or height; squares with their finite length and width but no height; and cubes with their finite length, width, and height. Take note that each dimension has its own measure. The deal with many fractals is that they can’t be measured very easily at all using these terms. Take Sierpinski’s triangle as an example.

Is this shape one- or two-dimensional? Many would say two-dimensional from first glance, but the same shape can be created using a line rather than a triangle.

So now it seems a bit more tricky. Is it one-dimensional since it can be made out of a line, or is it two-dimensional since it can be made out of a triangle? The answer is neither. The problem is that, if we were to treat it like a two-dimensional object, the measure of its dimension (area) would be zero. This is because we’ve technically taken away all of its area by taking out smaller and smaller triangles in every available space. On the other hand, if we were to treat it like a one-dimensional object, the measure of its dimension (length) would be infinity. This is because the line keeps getting longer and longer to stretch around each and every hole, of which there are an infinite number. So now we run into a problem: if it’s neither one- nor two-dimensional, then what is its dimensionality? To find out, we can use non-fractals
Measuring Integral Dimensions and Applying to Fractals
Let’s start with a one-dimensional line. The measure for a one-dimensional object is length. If we were to scale the line down by one-half, what is the fraction of the new length compared to the original length?

The new length of each line is one-half the original length.
Now let’s try the same thing for squares. The measure for a two-dimensional object is area. If we were to scale down a square by one-half (that is to say, if we were to divide the square’s length in half and divide its width in half), what is the fraction of the new area compared to the original area?

The new area of each square is one-quarter the original area.
If we were to try the same with cubes, the volume of each new cube would be one-eighth the original volume of a cube. These fractions provide us with a pattern we can work with.
In one dimension, the new length (one-half) is equal to the scaling factor (one-half) put to the first power (given by it being one-dimensional).
In two dimensions, the new area (one-quarter) is equal to the scaling factor (one-half) put to the second power (given by it being two-dimensional).
In three dimensions, the same pattern follows suit, in which the new volume (one-eighth) is equivalent to the scaling factor (one-half) put to the third power.
We can infer from this trend that the dimension of an object could be (not is) defined as the exponent fixed to the scaling factor of an object that determines the new measure of the object. To put it in mathematical terms:

Examples of this equation would include the one-dimensional line, the two-dimensional square, and the three-dimensional cube:
½ = ½^1
¼ = ½^2
1/8 = ½^3
Now this equation can be used to define the dimensionality of a given fractal. Let’s try Sierpinski’s Triangle again.

Here we can see that the triangle as a whole is made from three smaller versions of itself, each of which is scaled down by half of the original (this is proven by each side of the smaller triangles being half the length of the side of the whole triangle). So now we can just plug in the numbers to our equation and leave the dimension slot blank.
1/3 = ½^D
To solve for D, we need to know what power ½ must be put to in order to get 1/3. To do this, we can use logarithms (quick note: in this case, we can replace ½ with 2 and 1/3 with 3).
log_2(3) = roughly 1.585
So we can conclude that Sierpinski’s triangle is 1.585-dimensional. Now we can repeat this process with many other fractals. For example, this Sierpinski-esque square:

It’s made up of eight smaller versions of itself, each of which is scaled down by one-third. Plugging this into the equation, we get
1/8 = 1/3^D
log_3(8) = roughly 1.893
So we can conclude that this square fractal is 1.893-dimensional.
We can do this on this cubic version of it, too:

This cube is made up of 20 smaller versions of itself, each of which is scaled down by 1/3.
1/20 = 1/3^D
log_3(20) = roughly 2.727
So we can conclude that this fractal is 2.727-dimensional.
How is geometry entangled in the fabric of the Universe?
Geometry can be seen in action in all scales of the Universe, be it astronomical – in the orbital resonance of the planets for example, be it at molecular levels, in how crystals take their perfect structures. But going deeper and deeper into the fabric of the material world, we find that at quantum scale, geometry is a catalyst for many of the laws of quantum-physics and even definitions of reality.
Take for example the research made by Duncan Haldane, John Michael Kosterlitz and David Thouless, the winners of the 2016 Nobel prize in physics. By using geometry and topology they understood how exotic forms of matter take shape based on the effects of the quantum mechanics. Using techniques borrowed from geometry and topology, they studied the changes between states of matter (from plasma to gas, from gas to liquid and from liquid to solid) being able to generate a set of rules that explain different types of properties and behaviors of matter. Furthermore, by coming across a new type of symmetry patterns in quantum states that can influence those behaviors or even create new exotic types of matter, they provided new meaning and chance in using geometry as a study of “the real”.
If the Nobel Prize winners used abstract features of geometry to define physical aspects of matter, more tangible properties of geometry can be used to define other less tangible aspects of reality, like time or causality. In relativity, 3d space and 1d time become a single 4d entity called “space-time”, a dimension perceived through the eye of a space-time traveler. When an infinite number of travelers are brought into the equation, the numbers are adding up fast and, to see how for example, a space-time of a traveler looks for another traveler, we can use geometric diagrams of these equations. By tracing what a traveler “sees” in the space-time dimension and assuming that we all see the same speed of light, the intersection points of view between a stationary traveler and a moving one give a hyperbola that represents specific locations of space-time events seen by both travelers, no matter of their reference frame. These intersections represent a single value for the space-time interval, proving that the space-time dimension is dependent of the geometry given by the causality hyperbola.
If the abstract influence of geometry on the dimensions of reality isn’t enough, take for example one of the most interesting experiments of quantum-physics, the double-split experiment, were wave and particle functions are simultaneously proven to be active in light or matter. When a stream of photons is sent through a slit against a wall, the main expectation is that each photon will strike the wall in a straight line. Instead, the photons are rearranged by a specific type of patterns, called diffraction patterns, a behavior mostly visible for small particles like electrons, neutrons, atoms and small molecules, because of their short wavelength.

Two slits diffraction pattern by a plane wave. Animation by Fu-Kwun Hwang
The wave-particle duality is a handful, since it is a theory that has worked well in physics but with its meaning or representation never been satisfactorily resolved. Perhaps future experiments that will involve more abstract roles of geometry in quantum fields, like in the case of the Nobel prize winners, will develop new answers to how everything functions at these small scales of matter.
In this direction, Garret Lissi tries to explain how everything works, especially at quantum levels, by uniting all the forces of the Universe, its fibers and particles, into a 8 dimensions geometric structure. He suggests that each dot, or reference, in space-time has a shape, called fibre, attributed to each type of particle. Thus, a separate layer of space is created, parallel to the one we can perceive, given by these shapes and their interactions. Although the theory received also good reviews but also a widespread skepticism, its attempt to describe all known fundamental aspects of physics into one possible theory of everything is laudable. And proving that with laws and theories of geometry is one step closer to a Universal Geometry theory.



Geometry at work: Maxwell, Escher and Einstein
Maxwell’s diagram
from the 1821 “A Philosophical Magazine”, showing the rotative vortexes of electromagnetic forces, represented by hexagons and the inactive spaces between them
The impossible cube
invented in 1958, as an inspiration for his Belvedere litography.
Geometry of space-time
The three dimensions of space and the dimension of time give shape to the fourth, that of space-time.
A sand pendulum that creates a beautiful pattern only by its movement.
But why does the ellipse change shape?
The pattern gets smaller because energy is not conserved (and in fact decreases) in the system. The mass in the pendulum gets smaller and the center of mass lowers as a function of time. Easy as that, an amazing pattern arises through the laws of physics.
New Research Heading to Earth’s Orbiting Laboratory
It’s a bird! It’s a plane! It’s a…dragon? A SpaceX Dragon spacecraft is set to launch into orbit atop the Falcon 9 rocket toward the International Space Station for its 12th commercial resupply (CRS-12) mission August 14 from our Kennedy Space Center in Florida.

It won’t breathe fire, but it will carry science that studies cosmic rays, protein crystal growth, bioengineered lung tissue.

Here are some highlights of research that will be delivered:
I scream, you scream, we all scream for ISS-CREAM!
Cosmic Rays, Energetics and Mass, that is! Cosmic rays reach Earth from far outside the solar system with energies well beyond what man-made accelerators can achieve. The Cosmic Ray Energetics and Mass (ISS-CREAM) instrument measures the charges of cosmic rays ranging from hydrogen to iron nuclei. Cosmic rays are pieces of atoms that move through space at nearly the speed of light

The data collected from the instrument will help address fundamental science questions such as:
Do supernovae supply the bulk of cosmic rays?
What is the history of cosmic rays in the galaxy?
Can the energy spectra of cosmic rays result from a single mechanism?
ISS-CREAM’s three-year mission will help the scientific community to build a stronger understanding of the fundamental structure of the universe.
Space-grown crystals aid in understanding of Parkinson’s disease
The microgravity environment of the space station allows protein crystals to grow larger and in more perfect shapes than earth-grown crystals, allowing them to be better analyzed on Earth.

Developed by the Michael J. Fox Foundation, Anatrace and Com-Pac International, the Crystallization of Leucine-rich repeat kinase 2 (LRRK2) under Microgravity Conditions (CASIS PCG 7) investigation will utilize the orbiting laboratory’s microgravity environment to grow larger versions of this important protein, implicated in Parkinson’s disease.

Defining the exact shape and morphology of LRRK2 would help scientists to better understand the pathology of Parkinson’s and could aid in the development of therapies against this target.
Mice Help Us Keep an Eye on Long-term Health Impacts of Spaceflight
Our eyes have a whole network of blood vessels, like the ones in the image below, in the retina—the back part of the eye that transforms light into information for your brain. We are sending mice to the space station (RR-9) to study how the fluids that move through these vessels shift their flow in microgravity, which can lead to impaired vision in astronauts.

By looking at how spaceflight affects not only the eyes, but other parts of the body such as joints, like hips and knees, in mice over a short period of time, we can develop countermeasures to protect astronauts over longer periods of space exploration, and help humans with visual impairments or arthritis on Earth.
Telescope-hosting nanosatellite tests new concept
The Kestrel Eye (NanoRacks-KE IIM) investigation is a microsatellite carrying an optical imaging system payload, including an off-the-shelf telescope. This investigation validates the concept of using microsatellites in low-Earth orbit to support critical operations, such as providing lower-cost Earth imagery in time-sensitive situations, such as tracking severe weather and detecting natural disasters.

Sponsored by the ISS National Laboratory, the overall mission goal for this investigation is to demonstrate that small satellites are viable platforms for providing critical path support to operations and hosting advanced payloads.
Growth of lung tissue in space could provide information about diseases
The Effect of Microgravity on Stem Cell Mediated Recellularization (Lung Tissue) uses the microgravity environment of space to test strategies for growing new lung tissue. The cells are grown in a specialized framework that supplies them with critical growth factors so that scientists can observe how gravity affects growth and specialization as cells become new lung tissue.

The goal of this investigation is to produce bioengineered human lung tissue that can be used as a predictive model of human responses allowing for the study of lung development, lung physiology or disease pathology.
These crazy-cool investigations and others launching aboard the next SpaceX #Dragon cargo spacecraft on August 14. They will join many other investigations currently happening aboard the space station. Follow @ISS_Research on Twitter for more information about the science happening on 250 miles above Earth on the space station.
Watch the launch live HERE starting at 12:20 p.m. EDT on Monday, Aug. 14!
Make sure to follow us on Tumblr for your regular dose of space: http://nasa.tumblr.com

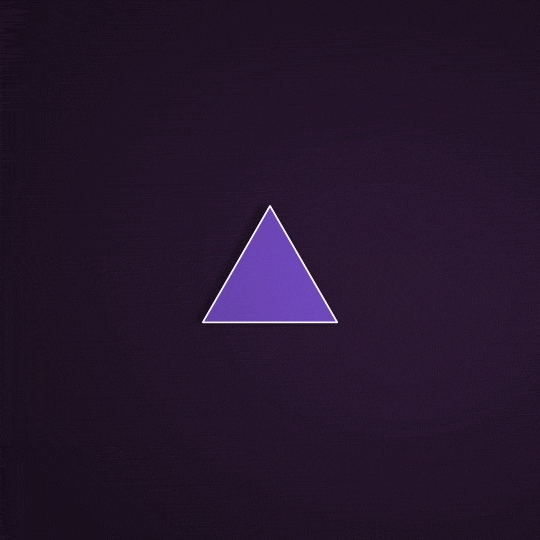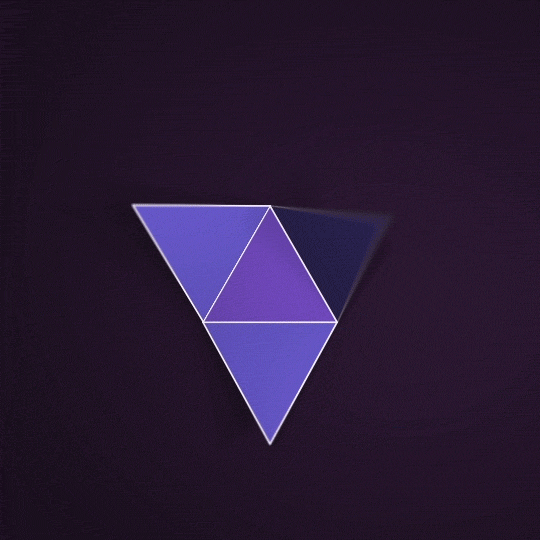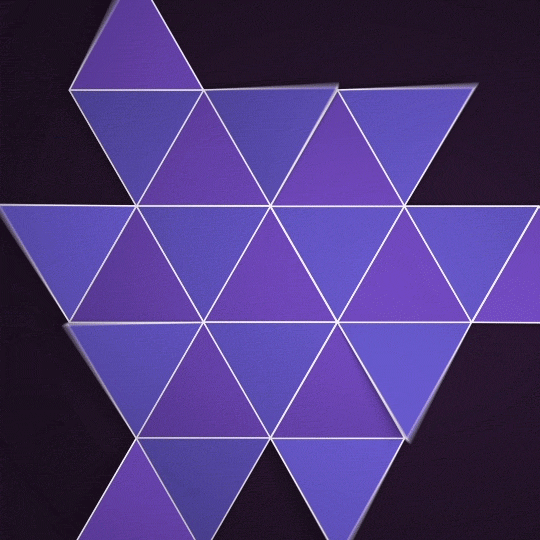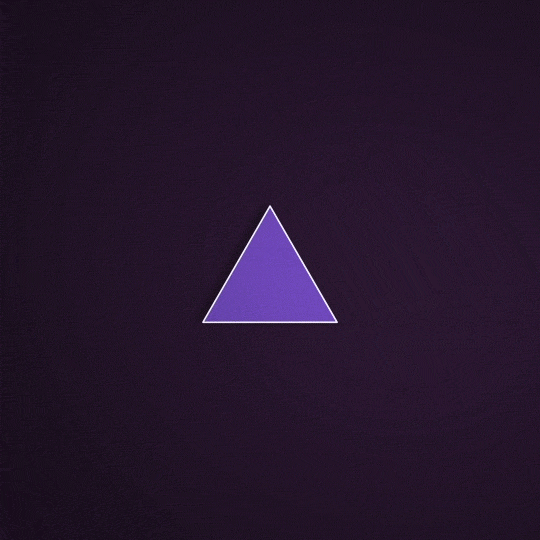

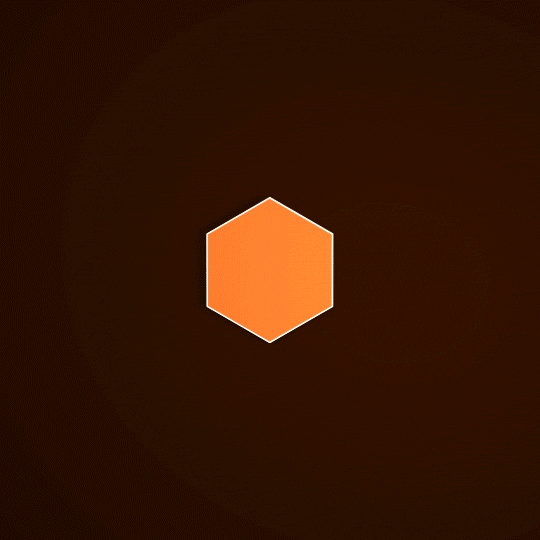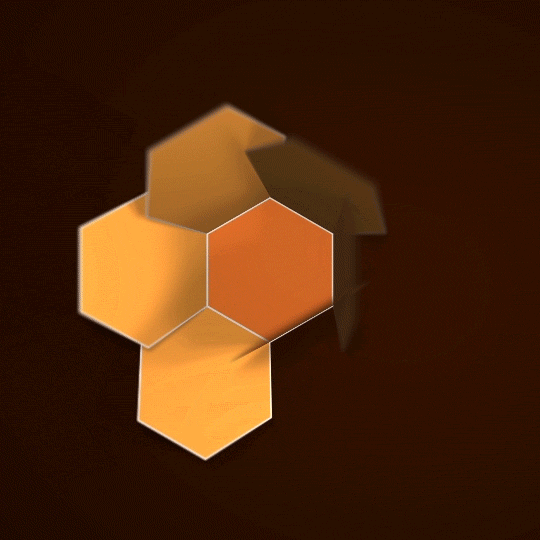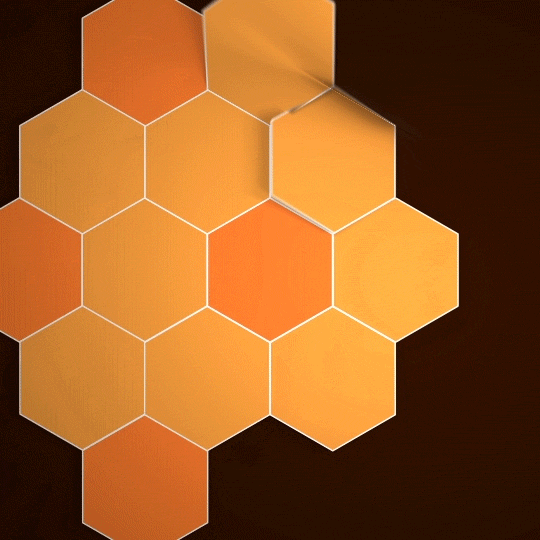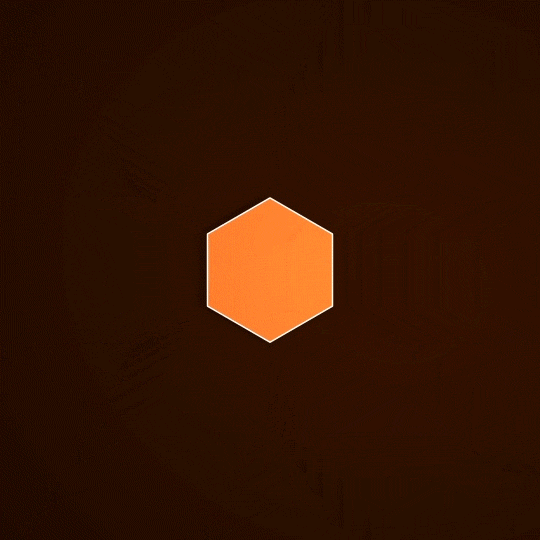

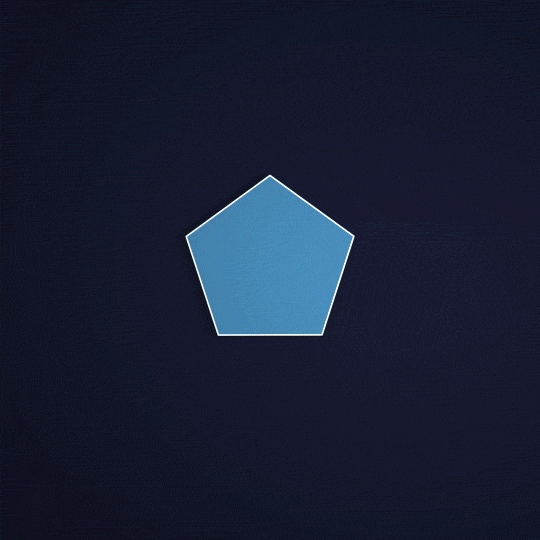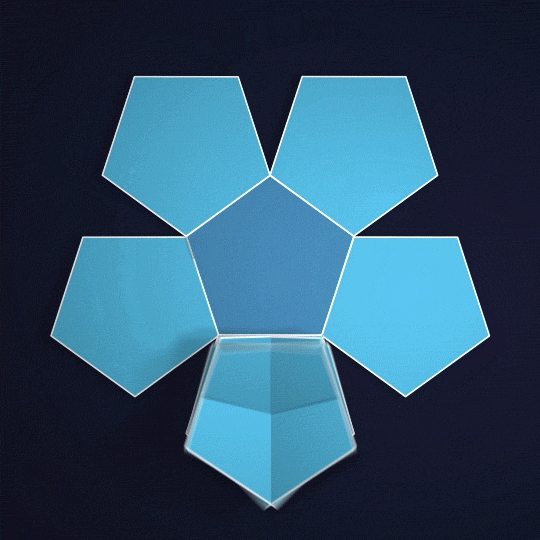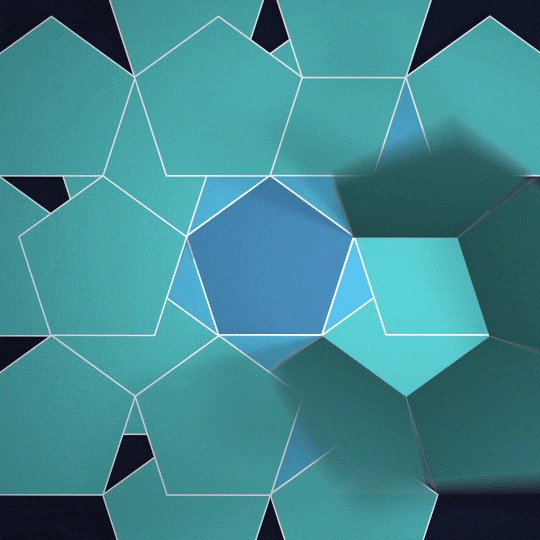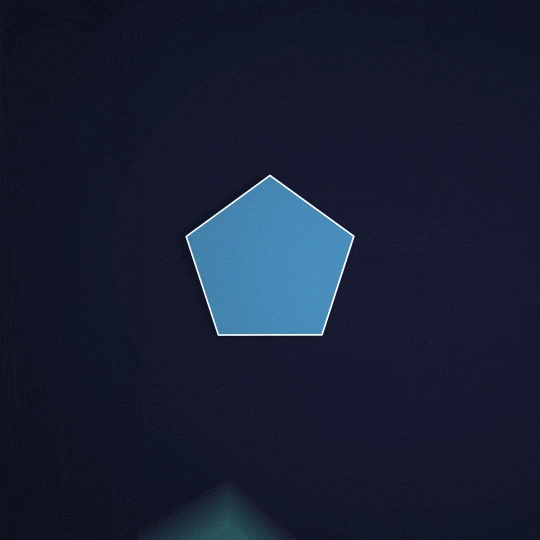

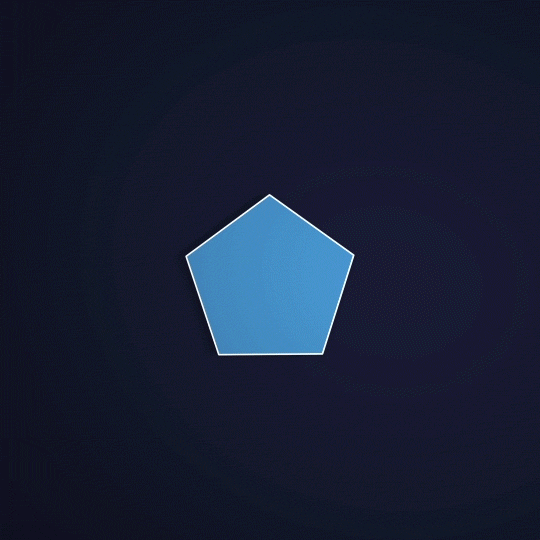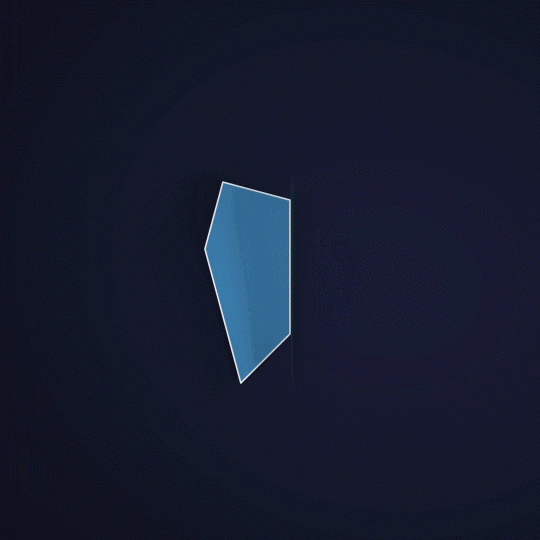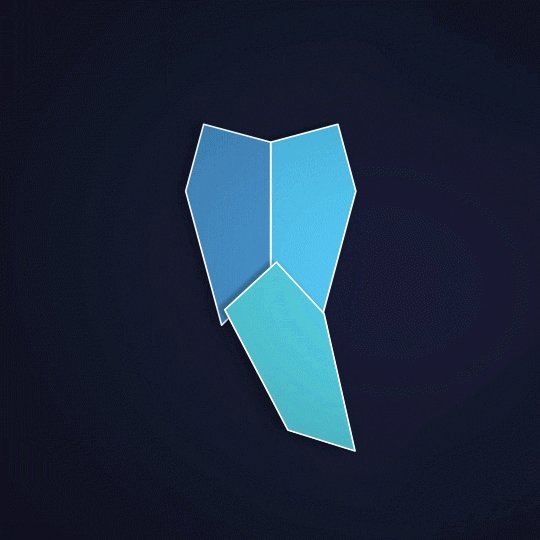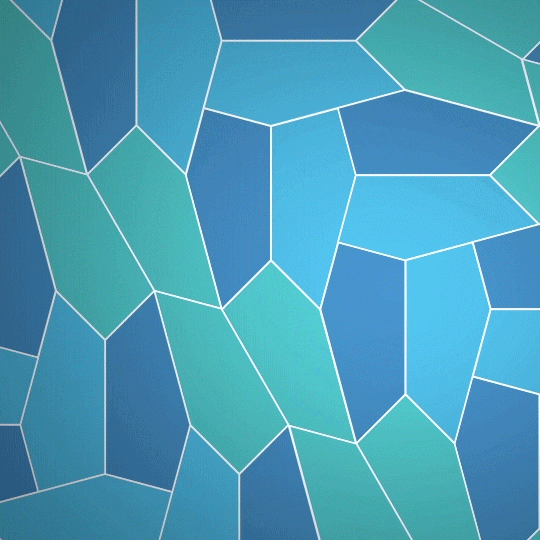
Big math news! It’s been thirty years since mathematicians last found a convex pentagon that could “tile the plane.” The latest discovery (by Jennifer McLoud-Mann, Casey Mann, and David Von Derau) was published earlier this month. Full story.
-
 gdel82 liked this · 1 month ago
gdel82 liked this · 1 month ago -
 eclectichellmouth reblogged this · 1 year ago
eclectichellmouth reblogged this · 1 year ago -
 bomberstillplaysvideogames liked this · 1 year ago
bomberstillplaysvideogames liked this · 1 year ago -
 glamtheobaupoma liked this · 1 year ago
glamtheobaupoma liked this · 1 year ago -
 requilune liked this · 1 year ago
requilune liked this · 1 year ago -
 highbottfeate liked this · 1 year ago
highbottfeate liked this · 1 year ago -
 neilfinnaesthetics liked this · 1 year ago
neilfinnaesthetics liked this · 1 year ago -
 theconfusedartist reblogged this · 2 years ago
theconfusedartist reblogged this · 2 years ago -
 schmaniel reblogged this · 2 years ago
schmaniel reblogged this · 2 years ago -
 thathalloweengal reblogged this · 2 years ago
thathalloweengal reblogged this · 2 years ago -
 rosebella2016 liked this · 3 years ago
rosebella2016 liked this · 3 years ago -
 fuckinganarwhal reblogged this · 3 years ago
fuckinganarwhal reblogged this · 3 years ago -
 sigma-umbra reblogged this · 3 years ago
sigma-umbra reblogged this · 3 years ago -
 sigma-umbra liked this · 3 years ago
sigma-umbra liked this · 3 years ago -
 imrryr liked this · 3 years ago
imrryr liked this · 3 years ago -
 afragmentcastadrift reblogged this · 3 years ago
afragmentcastadrift reblogged this · 3 years ago -
 afragmentcastadrift liked this · 3 years ago
afragmentcastadrift liked this · 3 years ago -
 polymorphousperve liked this · 3 years ago
polymorphousperve liked this · 3 years ago -
 ahellishhound reblogged this · 3 years ago
ahellishhound reblogged this · 3 years ago -
 lanternlightseer reblogged this · 3 years ago
lanternlightseer reblogged this · 3 years ago